Probability, Odds for Roulette Numbers, Dozens, Columns, Bets to Miss, Sleep, Skip
By Ion Saliu, Roulette Professor At-Large


Written by Ion Saliu on September 17, 2000; later updates.
In Reply to: Question on FFG and roulette dozens, posted by Noel on September 16, 2000.
: Ion,
: Here is my question to you at the "other" roulette message board (posted last Sept 15), and haven't heard a word since. Here's the excerpt...
"Anyway, I do believe in the concept of roulette number-sleeper showing up after a "considerable" number of spins, as in the case of your FFG. That's why I am trying to get "second" opinions from guys here at the roulette message board. BUT, sad to say, I don't believe in gambling system sellers much. Maybe the concept "try before you buy" is a more appealing approach for me (read posts below). =)
: Going back to your FFG (Fundamental Formula of Gambling), you said that "12-number spin will only encounter MORE than 18 LOSING roulette spins only in one 1000 spin-runs." But a check at the analysis at WIN-MAXX.COM roulette spins section yielded several incidents (more than 3, at least) of 18 or more SLEEPING roulette dozen/column on July 1-7, 2000 Spielbank Hamburg, of 2232 spins.
: Looking forward for your response."
: Noel
Noitser, I did respond to you and others on Mr. Oops' message board. Here are relevant excerpts, axiomatic one.
Steve:
• That's not the right interpretation overall.
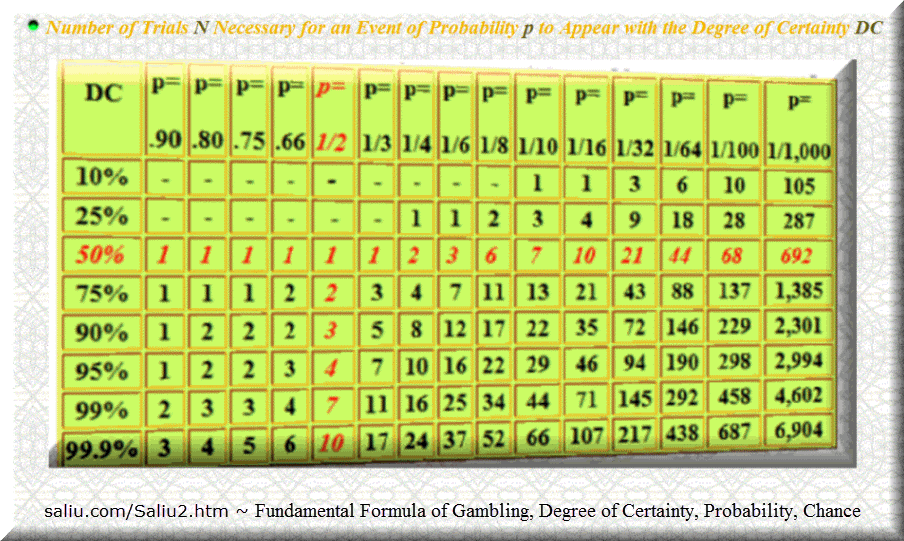
You are referring to the probability of N consecutive successes: (p) to the power of N. In your case, the probability of getting 18 consecutive 12-number-bet is (12/36) multiplied by itself 18 times. The result is so small that most calculators show zero or an error. The result is: 9.75 (to the power of power (–10)). That is, 0.0000000000975. Something like 1 in 10 billion.
Svitser, if you wanted the probability of 12/38 WITHIN 18 roulette spins, then you apply FFG. That is, at least once WITHIN 18 spins.
You might want also the probability of losing 18 times in a row. In this case, you consider the opposite of p, which is (1-p) = (1- 12/38) = 0.684. The power of 18 applied to .0.684 is 0.108%, or close to 1 in 1000 as calculated by FFG. It's still easier to lose 18 12-number bets in a row than winning 18 consecutive 12-number roulette bets! 10 million times easier!
I recommend you use my probability and statistical software SuperFormula. It calculates FFG and also the binomial distribution formula (BDF). You can use BDF to calculate the probability of consecutive successes or consecutive losses. Here is an example applied to the even-money bets. Let's say you want to play Red. The probability to win Red 10 consecutive times at roulette is: (18/38) to the power of 10 = 0.057% or 5.7 times in 10,000 roulette spins. The reverse, losing “Red” 10 times in a row (10 losses in 10 spins) is: (20/38) to the power of 10 = 0.16% or 1.6 times in 1,000 spins (clearly better than winning).
Paul:
FFG is NOT a gambling system per se, axiomatic colleague of mine. I emphasized that on my website. I have devised gambling systems based on FFG, but they go up to three levels deep in FFG. I do not offer any info in that direction, Plitser. I know, however, that players with mathematical inclination, or simply chess-like gamblers, have devised their own systems based on FFG. Frankly, I am not interested in casino gambling systems other than mine. There are many and complex enough strategies to consume a lot of my time.
Noel (Noitser), you said you encountered several streaks longer than 18 for 12-number roulette bets. The key point is ONE 12-NUMBER BET AT A TIME, not all of them. Take, for example, the 1-12 roulette dozen and see if it has 2 or more 19-or-longer losing streaks. Hardly so. The Fundamental Formula of Gambling does NOT imply that if a 12-number bet skipped 18 roulette spins it will necessarily come out the very next spin. It will in most cases, but NOT 100%. There is no 100% probability, not in gambling. Let's say you are soooo patient and wait for 18 losing streaks of a 12-number bet. If you place a bet that the 12-number will win the very next roulette spin, you will win in 999 out of 1000 SUCH cases.
Let's say you lost the first time. You are so patient and wait for the next 18-spin skip for the same 12-number bet. What if, this time, you'll increase your bet that the 12-number will hit the very next roulette spin? Your chance to lose is very, very slim. The chance to lose 18 in a row is 0.1% (or 0.001, we said 1 in 1000). To lose (2 times in a row (18-in-a-row)) is equal to 0.001 x 0.001 = 1 in 1 million. But, again, I am not recommending here a gambling system!
Paul (Plitser) is mathematically right to consider “staying with the trend”. I showed also on the lottery strategy page of my site (saliu.com/LottoWin.htm) that each lottery number repeats in a least 50% of the cases after a number of drawings EQUAL TO OR LESS THAN THE MEDIAN. That's also based on FFG. It is also validated by thousands of real-life drawings. You can check it at any lottery, at any time.
The same is true about the roulette spins. Just run my gambling software SuperRoulette. You will notice that each and every roulette number will repeat under the median in at least 50% of the cases. Doesn't it make more sense to play lottery or roulette numbers that show a current skip of UNDER the median? It makes a lot more sense than playing what you call “roulette sleepers”. You can see in the SuperRoulette reports that roulette numbers can sleep or miss or skip over 200 spins! But, again, I am not recommending here a roulette gambling system!
Mathematics is not everything in gambling: It's the ONLY thing (quoting Vince Lombardi). I remember also my excellent math teacher in high school. He started his probability classes with casino anecdotes. I remember he talk about a mathematician who did serious damage in Monte Carlo. I do not remember the details. I only remember at that time I committed myself to studying and mastering theory of probability.
Paulsy:
• You wrote:
“I guess that idea is not even mathematically sound but I always think there is a 50/50 chance no matter how long a run lasts that it could continue just as much as it could end”
So, Pytser, you have a run such as Heads 10 consecutive tosses, continues to 11 consecutive tosses, continues to 12 tosses, … continues all the way to 100 consecutive tosses … and can continue like that for ever … You are looking at two different events, very different from each other. Indeed, every time you toss a coin, the probability of Heads is 1/2, always 1 in 2. Then, there is another event, such as 10 heads in a row. Or, there is another event, such as 5 heads in 10 tosses. This is the stuff the binomial distribution probability is made of.
One more thing, axiomatic one: Theory of probability is the science of streaks. Run that great piece of software, Streaks, and you'll be convinced.
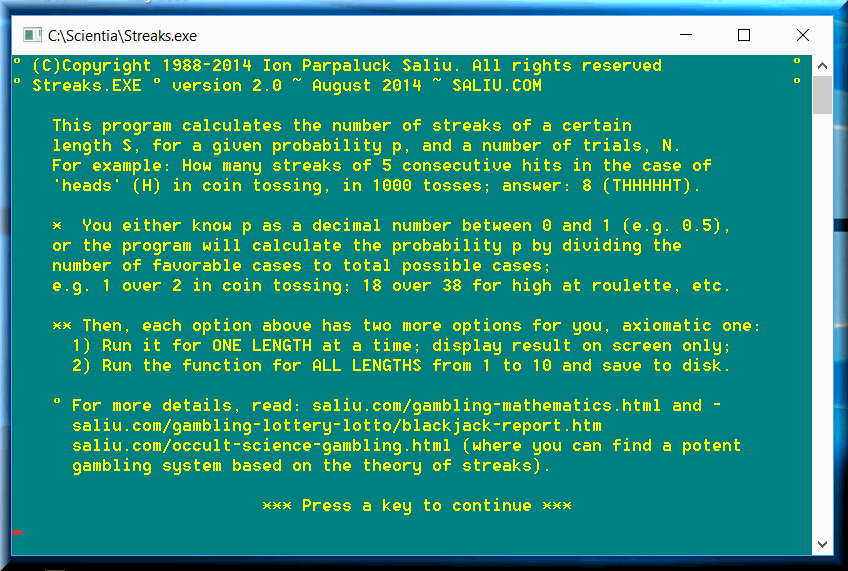
Why don't you try for yourself to see that the streak frequency is never, ever equally distributed. Take a coin and toss it 1024 times, axiomatic one. You will notice that 1-Heads occurred around 128 times; 2-Heads-in-a-row occurred 64 times; 3-Heads-in-a-row occurred 32 times; “4-Heads-in-a-row” occurred 16 times; … 8-or-more-Heads-in-a-row occurred only 1 time. (Similar numbers occur for Tails.)
Why aren't the streaks equal? Why are so rare streaks of 10 or longer (let alone 100-long streaks!) You can repeat this experiment for the rest of your life and the numbers shown above will occur consistently. That is, the results will be very close to the theoretical values shown above. As a matter of fact, the values will be extremely close PERCENTAGE-WISE the more tosses you do (10,000 or more).
See also the roulette links and resources that follow on this page. Not to mention how greatly I improved my roulette software and systems. Everything is now bundled in a great roulette package: BrightR.
Follow-up:
Roulette: Software, Systems, Super Roulette Strategy
See a comprehensive directory of the pages and materials on the subject of roulette, software, systems, and the Super Strategy.

