
Fundamental Formula of Gambling (FFG): Theory of Probability, Mathematics, Degree of Certainty, Chance
★ ★ ★ ★ ★
By Ion Saliu,
Founder of Gambling Mathematics, Founder of Probability Theory of Life



- First captured by the WayBack Machine (web.archive.org) on April 17, year of grace 2000.
- Presenting the most astonishing formula in gambling mathematics, probability theory at large, widely known now as FFG. Indeed, it is the most essential formula of theory of probability. This formula was directly derived from the most fundamental formula of probability: Number of favorable cases, n, over Total possible cases, N: n / N. Abraham de Moivre, a French/English-refugee mathematician and philosopher discovered the first steps of this formula that explains the Universe the best. I believe Monsieur de Moivre was frightened by the implications of finalizing such formula would have led to: The absurdity of the concept of God. I did finalize the formula, for the risks in my lifetime pale by comparison to the eighteenth century. God, no doubt, represents the limit of mathematical absurdity, therefore of all Absurdity.
And thusly we discovered here the much-feared mathematical concept of Degree of Certainty, DC. I introduced the DC concept in the year of grace 1997, or 1997+1 years after tribunicia potestas were granted to Octavianus Augustus (the point in time humans started the year count of Common Era, still in use). The Internet search on Degree of Certainty, DC yielded one and only one result in 1998: This very Web page (zero results in 1997, for DC was introduced in December of that glorious year, with some beautiful snowy days
just before the Global Warming debate started
) For we shall always be mindful that nothing comes in absolute certainty; everything comes in degrees of certainty Never zero, Never absolutely. Never say never; never say forever!
- The degree of certainty DC rises exponentially with the increase in the number of trials N while the probability p is always the same or constant.
- DC = 1 (1 p) ^ N
- Simultaneously, the opposite event, the losing chance, decreases exponentially with an increase in the number of trials. That's the fundamental reason why the infamous gambler's fallacy is an obvious absurdity.
1. Theory of Probability Leading to the Fundamental Formula of Gambling
 It has become common sense the belief that persistence leads to success. It might be true for some life situations, sometimes. It is never true, however, for gambling and games of chance in general. Actually, in gambling persistence leads to inevitable bankruptcy. I can prove this universal truth mathematically. I will not describe the entire scientific process, since it is rather complicated for all readers but a few. The algorithm consists of four phases: win N consecutive draws (trials); lose N consecutive trials; not to lose N consecutive draws; win within N consecutive trials.
It has become common sense the belief that persistence leads to success. It might be true for some life situations, sometimes. It is never true, however, for gambling and games of chance in general. Actually, in gambling persistence leads to inevitable bankruptcy. I can prove this universal truth mathematically. I will not describe the entire scientific process, since it is rather complicated for all readers but a few. The algorithm consists of four phases: win N consecutive draws (trials); lose N consecutive trials; not to lose N consecutive draws; win within N consecutive trials.
• I will simplify the discourse to its essentials. You may want to know the detailed procedure leading to this numerical relation. Read: Mathematics of the Fundamental Formula of Gambling (FFG).
•• Visit the software download site (in the footer of this page) to download SuperFormula; the extraordinary software automatically does all FFG calculations, plus several important statistics and probability functions.
The probability and statistical program allows you to calculate the number of trials N for any degree of certainty DC. Plus, you can also calculate the very important binomial distribution formula (BDF) and binomial standard deviation (BSD), plus dozens of statistics and probability functions.

Let's suppose I play the 3-digit lottery game (pick 3). The game has a total of 1,000 combinations. Thus, any particular pick-3 combination has a probability of 1 in 1,000 (we write it 1/1,000). I also mention that all combinations have an equal probability of appearance. Also important - and contrary to common belief the past draws do count in any game of chance. Pascal demonstrated that truth hundreds of years ago.
Evidently, the same-lotto-game combinations have an equal probability, p always the same but they appear with different statistical frequencies. Standard deviation plays an essential role in random events. The Everything, that is; for everything is random. Most people don't comprehend the concept of all-encompassing randomness because phenomena vary in the particular probability, p, and specific degree of certainty, DC, directly influenced by the number of trials, N. Please read an important article here: Combination 1 2 3 4 5 6: Probability and Reality. A 6-number lotto combination such as 1 2 3 4 5 6 should have appeared by now at least once, considering all the drawings in all lotto-6 games ever played in the world. It hasn't come out and will not appear in my lifetime... I bet on it... even if I live 100 years after 2060, when Isaac Newton calculated that the world would end based on his mathematical interpretation of the Bible! (Newton and Einstein belong to the special class of the most intelligent mystics in human and natural history.) Instead, other lotto combinations, with a more natural standard devi(l)ation (yes, deviation), will repeat in the same frame of time.
As soon as I choose a combination to play (for example 2-1-4) I can't avoid asking myself: "Self, how many drawings do I have to play so that there is a 99.9% degree of certainty my combination of 1/1,000 probability will come out?"
My question dealt with three elements:
• degree of certainty that an event will appear, symbolized by DC
• probability of the event, symbolized by p
• number of trials (events), symbolized by N
I was able to answer such a question and quantify it in a mathematical expression (logarithmic) I named the Fundamental Formula of Gambling (FFG):
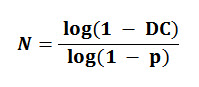
The Fundamental Formula of Gambling is an historic discovery in theory of probability, theory of games, and gambling mathematics. The formula offers an incredibly real and practical correlation with gambling phenomena. As a matter of fact, FFG is applicable to any sort of highly randomized events: lottery, roulette, blackjack, horse racing, sports betting, even stock trading. By contrast, what they call theory of games is a form of vague mathematics: The formulae are barely vaguely correlated with real life.

2. The Fundamental Table of Gambling (FTG)
Substituting DC and p with various values, the formula leads to the following, very meaningful and useful table. You may want to keep it handy and consult it especially when you want to bet big (as in a casino).
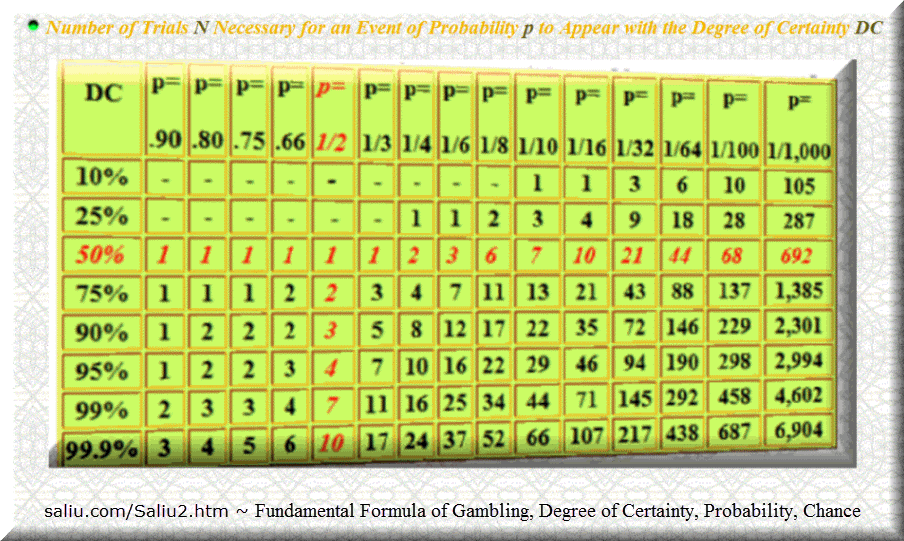
Let's try to make sense of those numbers. The easiest to understand are the numbers in the column under the heading p=1/2. It analyzes the coin tossing game of chance. There are 2 events in the game: heads and tails. Thus, the individual probability for either event is p = 1/2. Look at the row 50%: it has the number 1 in it. It means that it takes 1 event (coin toss, that is) in order to have a 50-50 chance (or degree of certainty of 50%) that either heads or tails will come out. More explicitly, suppose I bet on heads. My chance is 50% that heads will appear in the 1st coin toss. The chance or degree of certainty increases to 99.9% that heads will come out within 10 tosses!
Even this easiest of the games of chance can lead to sizable losses. Suppose I bet $2 before the first toss. There is a 50% chance that I will lose. Next, I bet $4 in order to recuperate my previous loss and gain $2. Next, I bet $8 to recuperate my previous loss and gain $2. I might have to go all the way to the 9th toss to have a 99.9% chance that, finally, heads came out! Since I bet $2 and doubling up to the 9th toss, two to the power of 9 is 512. Therefore, I needed $512 to make sure that I am very, very close to certainty (99.9%) that heads will show up and I win . . . $2!
Very encouraging, isn't it? Actually, it could be even worse: It might take 10 or 11 tosses until heads appear! This dangerous form of betting is called a Martingale system. You must know how to do it study this book thoroughly and grasp the new essential concepts: Number of trials N and especially the Degree of Certainty DC (in addition to the probability p).
Most people still confuse probability for degree of certainty...or vice versa. Probability in itself is an abstract, lifeless concept. Probability comes to life as soon as we conduct at least one trial. The probability and degree of certainty are equal for one and only one trial (just the first one...ever!) After that quasi-impossible event (for coin tossing has never been stopped after one flip by any authority), the degree of certainty, DC, rises with the increase in the number of trials, N, while the probability, p, always stays constant. No one can add faces to the coin or subtract faces from the die, for sure and undeniably. But each and every one of us can increase the chance of getting heads (or tails) by tossing the coin again and again (repeat of the trial).
Normally, though, you will see that heads (or tails) will appear at least once every 3 or 4 tosses (the DC is 90% to 95%). Nevertheless, this game is too easy for any player with a few thousand dollars to spare. Accordingly, no casino in the world would implement such a game. Any casino would be a guaranteed loser in a matter of months! They need what is known as house edge or percentage advantage. This factor translates to longer losing streaks for the player, in addition to more wins for the house! Also, the casinos set limits on maximum bets: the players are not allowed to double up indefinitely.
A few more words on the house advantage (HA). The worst type of gambling for the player is conducted by state lotteries. In the digit lotteries, the state commissions enjoy typically an extraordinary 50% house edge!!! That's almost 10 times worse than the American roulette -- considered by many a suckers' game! (But they don't know there is more to the picture than meets the eye!)
In order to be as fair as the roulette, the state lotteries would have to pay $950 for a $1 bet in the 3-digit game. In reality, they now pay only $500 for a $1 winning bet!!! Remember, the odds are 1,000 to 1 in the 3-digit game...
If private organizations, such as the casinos, would conduct such forms of gambling, they would surely be outlawed on the grounds of extortion! In any event, the state lotteries defy all anti-trust laws: they do not allow the slightest form of competition! Nevertheless, the state lotteries may conduct their business because their hefty profits serve worthy social purposes (helping the seniors, the schools, etc.) Therefore, lotteries are a form of taxation - the governments must tell the truth to their constituents...

3. Fundamental Formula of Gambling: Games Other Than Coin Tossing
Dice rolling is a more difficult game and it is illustrated in the column p=1/6. I bet, for example, on the 3-point face. There is a 50% chance (DC) that the 3-point face will show up within the first 3 rolls. It will take, however, 37 rolls to have a 99.9% certainty that the 3-point face will show up at least once. If I bet the same way as in the previous case, my betting capital should be equal to 2 to the power of 37! It's already astronomical and we are still in easy-gambling territory!
Let's go all the way to the last column: p=1/1,000. The column illustrates the well-known3-digit lottery game. It is extremely popular and supposedly easy to win. Unfortunately, most players know little, if anything, about its mathematics. Let's say I pick the number 2-1-4 and play it every drawing. I only have a 10% chance (DC) that my pick will come out winner within the next 105 drawings!
The degree of certainty DC is 50% that my number will hit within 692 drawings! Which also means that my pick will not come out before I play it for 692 drawings. So, I would spend $692 and maybe I win $500! If the state lotteries want to treat their customers (players like you and me) more fairly, they should pay $690 or $700 for a $1 winning ticket. That's where the 50-50 chance line falls.
In numerous other cases it's even worse. I could play my daily-3 number for 4,602 drawings and, finally, win. Yes, it is almost certain that my number will come out within 4,602 or within 6,904 drawings! Real life case: Pennsylvania State Lottery has conducted over 6,400 drawings in the pick3 game. The number 2,1,4 has not come out yet!...
All lottery cases and data do confirm the theory of probability and the formula of bankruptcy... I mean of gambling! By the way, it is almost certain (99.5% to 99.9%) that the number 2-1-4 will come out within the next 400-500 drawings in Pennsylvania lottery. But nothing is 100% certain, not even... 99.99%!
We don't need to analyze the lotto games. The results are, indeed, catastrophic. If you are curious, simply multiply the numbers in the last column by 10,000 to get a general idea. To have a 99.9% degree of certainty that your lotto (pick-6) ticket (with 6 numbers) will come out a winner, you would have to play it for over 69 million consecutive drawings! At a pace of 100 drawings a year, it would take over 690,000 years!

4. Ion Saliu's Paradox or Problem of N Trials
We can express the probability as p = 1/N; e.g. the probability of getting one point face when rolling a die is 1 in 6 or p = 1/6; the probability of getting one roulette number is 1 in 38 or p = 1/38. It is common sense that if we repeat the event N times we expect one success. That might be true for an extraordinarily large number of trials. If we repeat the event N times, we are NOT guaranteed to win. If we play roulette 38 consecutive spins, the chance to win is significantly less than 1!
A step in the Fundamental Formula of Gambling leads to this relation:
DC = 1 1/e
The limit 1 1/e is approximately 0.632120558828558...
I tested for N = 100,000,000
N = 500,000,000
N = 1,000,000,000 (one billion) trials. The results ever so slightly decrease, approaching the limit
but never surpass the limit!
When N = 100,000,000, then DC = .632120560667764...
When N = 1,000,000,000, then DC = .63212055901829...
(Calculations performed by SuperFormula, option C = Degree of Certainty (DC), then option 1 = Degree of Certainty (DC), then option 2 = The program calculates p.)
If the probability is 1/N and we repeat the event N times, the degree of certainty is 1 (1/e), when N tends to infinity. I named this relation: Ion Saliu Paradox of N Trials. Read more on my Web pages: Theory of Probability: Best introduction, formulae, algorithms, software and Mathematics of Fundamental Formula of Gambling.

5. Practical Dimension of Fundamental Formula of Gambling
There is more info on this topic on the next page. It reveals the dark side of the Moon, so to speak. The governments hide the truth when it comes to telling it all; and the Internet is incredibly prone to fraudulent gambling. Read revealing facts: Lottery, Lotto, Gambling, Odds, House Edge, Fraud.
The Fundamental Formula of Gambling does not explicitly or implicitly serve as a gambling system. It represents pure mathematics. Users who apply the numerical relations herein to their own gambling systems do so at their risk entirely. I, the author, do apply the formula to my gambling and lottery systems. I will show you how to use the gambling formula, my application MDIEditor and Lotto and the lotto systems that come with the application. I will put everything in a winning lotto strategy that targets the third prize in lotto games (4 out of 6).
At later times, I also released gambling systems, strategies for:
Is it all? Probably you'll find a lot more on this extraordinary site
|
 Read Ion Saliu's first book in print: Probability Theory, Live!
Read Ion Saliu's first book in print: Probability Theory, Live!
~ Discover profound philosophical implications of the Fundamental Formula of Gambling (FFG), including mathematics, probability, formula, gambling, lottery, software, degree of certainty, randomness.

6. Resources in Theory of Probability, Mathematics, Statistics, Combinatorics, Software
See a comprehensive directory of the pages and materials on the subject of theory of probability, mathematics, statistics, combinatorics, plus software.
- Theory of Probability: Best introduction, formulae, algorithms, software.
- Bayes Theorem, Conditional Probabilities, Simulation; Relation to Ion Saliu's Paradox.
- Standard Deviation: Theory, Algorithm, Software.
Standard deviation: Basics, mathematics, statistics, formula, software, algorithm.
- Standard Deviation, Gauss, Normal, Binomial, Distribution
Calculate: Median, degree of certainty, standard deviation, binomial, hypergeometric, average, sums, probabilities, odds.
- Combinatorial Mathematics: Calculate, Generate Exponents, Permutations, Sets, Arrangements, Combinations for Any Numbers and Words.
- Caveats in Theory of Probability.
- The Best Strategy for Lottery, Gambling, Sports Betting, Horse Racing, Blackjack, Roulette.
- Birthday Paradox Probability Formula, Odds of Duplication, Software.
- Monty Hall Paradox, 3-Door Problem, Probability Paradoxes.
- Couple Swapping, Husband Wife Swapping, Probability, Odds.
- Download Probability, Mathematics, Statistics Software.

Home | Search | New Writings | Ion Saliu | Odds, Generator | Contents | Forums | Sitemap



 It has become common sense the belief that persistence leads to success. It might be true for some life situations, sometimes. It is never true, however, for gambling and games of chance in general. Actually, in gambling persistence leads to inevitable bankruptcy. I can prove this universal truth mathematically. I will not describe the entire scientific process, since it is rather complicated for all readers but a few. The algorithm consists of four phases: win N consecutive draws (trials); lose N consecutive trials; not to lose N consecutive draws; win within N consecutive trials.
It has become common sense the belief that persistence leads to success. It might be true for some life situations, sometimes. It is never true, however, for gambling and games of chance in general. Actually, in gambling persistence leads to inevitable bankruptcy. I can prove this universal truth mathematically. I will not describe the entire scientific process, since it is rather complicated for all readers but a few. The algorithm consists of four phases: win N consecutive draws (trials); lose N consecutive trials; not to lose N consecutive draws; win within N consecutive trials.

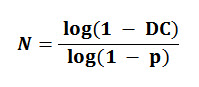

 Read Ion Saliu's first book in print:
Read Ion Saliu's first book in print: